How To Find The Activation Energy Of A Reaction

6.2.iii.iii: The Arrhenius Law - Activation Energies

Last updated
Save equally PDF

Folio ID: 1444

All molecules possess a certain minimum amount of energy. The free energy can exist in the course of kinetic energy or potential energy. When molecules collide, the kinetic energy of the molecules can exist used to stretch, bend, and ultimately break bonds, leading to chemical reactions. If molecules motion too slowly with niggling kinetic energy, or collide with improper orientation, they practise non react and only bounce off each other. Still, if the molecules are moving fast enough with a proper collision orientation, such that the kinetic free energy upon collision is greater than the minimum energy barrier, then a reaction occurs. The minimum energy requirement that must be met for a chemical reaction to occur is chosen the activation energy, \(E_a\).

Effigy 1: In Greek mythology Sisyphus was punished past existence forced gyre an immense boulder upwardly a colina, only to scout it roll dorsum downwardly, and to repeat this action forever. If this were a chemic reaction, then it would never be observed, since the reactants must overcome the energy barrier to get to the other side (products).

The reaction pathway is similar to what happens in Effigy i. To get to the other end of the road, an object must roll with enough speed to completely curlicue over the hill of a certain acme. The faster the object moves, the more than kinetic energy information technology has. If the object moves too slowly, it does not have plenty kinetic free energy necessary to overcome the barrier; as a event, it eventually rolls back down. In the same way, there is a minimum amount of energy needed in order for molecules to pause existing bonds during a chemical reaction. If the kinetic free energy of the molecules upon collision is greater than this minimum energy, then bond breaking and forming occur, forming a new production (provided that the molecules collide with the proper orientation).

Figure two: Reaction coordinate diagram for the bimolecular nucleophilic substitution (\(S_N2\)) reaction betwixt bromomethane and the hydroxide anion. from Wikipedia.

The activation energy (\(E_a\)), labeled \(\Delta{G^{\ddagger}}\) in Figure 2, is the energy difference between the reactants and the activated circuitous, also known as transition state. In a chemical reaction, the transition state is defined as the highest-energy state of the system. If the molecules in the reactants collide with enough kinetic energy and this energy is higher than the transition country energy, then the reaction occurs and products class. In other words, the higher the activation free energy, the harder it is for a reaction to occur and vice versa.

Effects of Enzymes on Activation Free energy

Nonetheless, if a catalyst is added to the reaction, the activation energy is lowered because a lower-free energy transition land is formed, equally shown in Effigy iii. Enzymes tin exist thought of equally biological catalysts that lower activation energy. Enzymes are proteins or RNA molecules that provide alternate reaction pathways with lower activation energies than the original pathways. Enzymes affect the rate of the reaction in both the forward and contrary directions; the reaction proceeds faster considering less energy is required for molecules to react when they collide. Thus, the charge per unit constant (grand) increases.

Figure \(\PageIndex{1}\): Lowering the Activation Energy of a Reaction past a Goad. This graph compares potential energy diagrams for a single-step reaction in the presence and absence of a catalyst. The just upshot of the catalyst is to lower the activation energy of the reaction. The catalyst does not touch on the energy of the reactants or products (and thus does non touch on ΔE). (CC BY-NC-SA; anonymous)

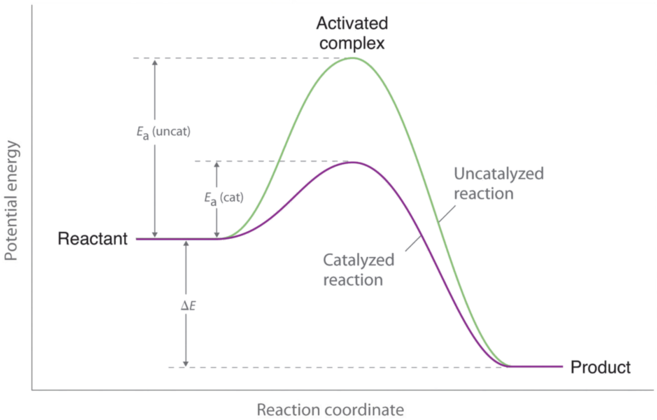
Equally indicated past Figure 3 above, a catalyst helps lower the activation energy barrier, increasing the reaction charge per unit. In the case of a biological reaction, when an enzyme (a grade of catalyst) binds to a substrate, the activation free energy necessary to overcome the barrier is lowered, increasing the rate of the reaction for both the forward and reverse reaction. See below for the effects of an enzyme on activation free energy.

Catalysts exercise not just reduce the energy barrier, but induced a completely different reaction pathways typically with multiple free energy barriers that must be overcome. For example:

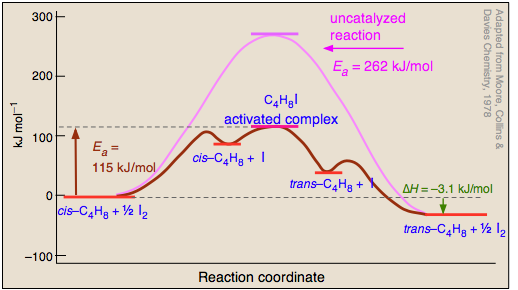
The Iodine-catalyzed cis-trans isomerization

Activation Enthalpy, Entropy and Gibbs Energy

In thermodynamics, the modify in Gibbs energy, ΔG, is defined equally:

\[ \Delta Thou = \Delta H - T \Delta S \characterization{i} \]

where

ΔG = alter in Gibbs free energy of the reaction
ΔH = change in enthalpy
ΔS = change in entropy

\( \Delta G^o \) is the change in Gibbs free energy when the reaction happens at Standard Land (i atm, 298 Thousand, pH 7). To calculate a reaction's modify in Gibbs free energy that did non happen in standard state, the Gibbs complimentary energy equation tin can be written as:

\[ \Delta Chiliad = \Delta G^o + RT\ \ln K \characterization{ii}\]

where

ΔG is alter in Gibbs free free energy of the reaction
ΔG^o is the standard Gibbs gratuitous energy
R is the Platonic Gas constant (viii.314 J/mol K)
\(K\) is the equilibrium constant

When the reaction is at equilibrium, \( \Delta G = 0\). The equation above becomes:

\[ 0 = \Delta G^o + RT\ln K \]

Solve for ΔG^o:

\[ \Delta Yard^o = -RT \ln K \]

Similarly, in transition land theory, the Gibbs energy of activation, \( \Delta G ^{\ddagger} \), is defined by:

\[ \Delta G ^{\ddagger} = -RT \ln Thou^{\ddagger} \label{3}\]

and

\[ \Delta G ^{\ddagger} = \Delta H^{\ddagger} - T\Delta S^{\ddagger}\label{4} \]

where

\( \Delta G^{\ddagger} \) is the Gibbs energy of activation
\( \Delta H^{\ddagger} \) is the enthalpy of activation
\( \Delta Southward^{\ddagger} \) is the entropy of activation

Combining equations 3 and iv and then solve for \(\ln K^{\ddagger}\) nosotros have the Eyring equation:

\[ \ln K^{\ddagger} = -\dfrac{\Delta H^{\ddagger}}{RT} + \dfrac{\Delta S^{\ddagger}}{R} \]

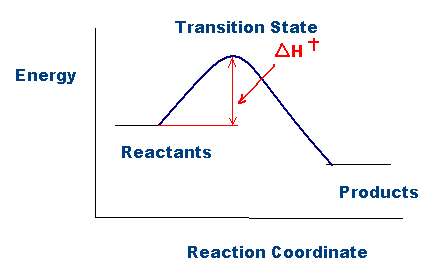
As shown in the effigy in a higher place, activation enthalpy, \(\Delta{H}^{\ddagger} \), represents the difference in energy between the ground state and the transition land in a chemical reaction. The college the activation enthalpy, the more energy is required for the products to form. Note that this activation enthalpy quantity, \( \Delta{H}^{\ddagger} \), is analogous to the activation energy quantity, E_a, when comparing the Arrhenius equation (described below) with the Eyring equation:

\[E_a = \Delta{H}^{\ddagger} + RT\]

In general, a reaction gain faster if E_a and \(\Delta{H}^{\ddagger} \) are small. Conversely, if E_a and \( \Delta{H}^{\ddagger} \) are big, the reaction rate is slower.

Calculation of E_a using Arrhenius Equation

Equally temperature increases, gas molecule velocity also increases (according to the kinetic theory of gas). This is also truthful for liquid and solid substances. The (translational) kinetic energy of a molecule is proportional to the velocity of the molecules (KE = 1/2 mv^two). Therefore, when temperature increases, KE besides increases; every bit temperature increases, more molecules have higher KE, and thus the fraction of molecules that have high enough KE to overcome the energy bulwark also increases.

The fraction of molecules with energy equal to or greater than E_a is given past the exponential term \(e^{\frac{-E_a}{RT}}\) in the Arrhenius equation:

\[k = Ae^{\frac{-E_a}{RT}} \label{5}\]

one thousand is the rate constant
E_a is the activation energy
R is the gas constant
T is temperature in Kelvin
A is frequency gene constant or also known every bit pre-exponential factor or Arrhenius factor. It indicates the rate of collision and the fraction of collisions with the proper orientation for the reaction to occur.

Taking the natural log of both sides of Equation \(\ref{5}\) yields the following:

\[\ln m = \ln A - \frac{E_a}{RT} \label{half-dozen}\]

Equation \(\ref{iv}\) has the linear form y = mx + b. Graphing ln g vs 1/T yields a directly line with a slope of -E_a/R and a y-intercept of ln A., as shown in Figure 4.

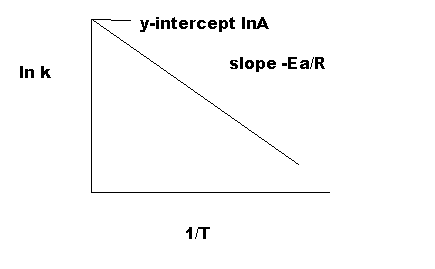
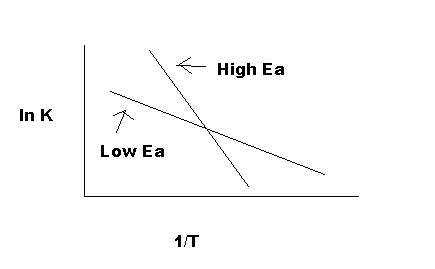
Equally indicated in Effigy 5, the reaction with a higher E_a has a steeper gradient; the reaction charge per unit is thus very sensitive to temperature change. In contrast, the reaction with a lower E_a is less sensitive to a temperature change. Because radicals are extremely reactive, East_a for a radical reaction is 0; an arrhenius plot of a radical reaction has no gradient and is independent of temperature.

The activation energy can too exist calculated directly given two known temperatures and a rate constant at each temperature. Using Equation (2), suppose that at two different temperatures T_one and T₂, reaction rate constants k_i and thousand₂:

\[\ln\; k_1 = - \frac{E_a}{RT_1} + \ln A \label{7} \]

and

\[\ln\; k_2 = - \frac{E_a}{RT_2} + \ln A \label{8} \]

Subtract \(ln\; k_2\) from \(ln\; k_1\):

\[ \ln\; k_1 - \ln\; k_2 = \left (- \dfrac{E_a}{RT_1} + \ln A \right ) - \left(- \dfrac{E_a}{RT_2} + \ln A \correct) \label{9} \]

After rearrangement:

\[ \ln \left (\dfrac{k_1}{k_2} \correct ) = \left(\dfrac{1}{T_2} - \dfrac{i}{T_1}\right)\dfrac{E_a}{R} \label{10} \]

Questions

Given that the rate constant is 11 M^-ones^-one at 345 K and the pre-exponential factor is 20 1000^-anes^-1, calculate the activation energy.
If a reaction'southward rate abiding at 298K is 33 M^-1s^-1 and 45 G^-1s^-one at 675 1000, what is the activation energy?
What is the Gibbs free free energy change at the transition state when ΔH at the transition state is 34 kJ/mol and ΔS at transition state is 66 J/mol at 334K?
What is the Activation Free energy of a reverse reaction at 679K if the forward reaction has a charge per unit abiding of 50M^-1s^-1, a pre-exponential cistron of 30M^-anes^-i, and a ΔH of reaction of 23 kJ/mol?
Enzymes lower activation energy, and thus increase the rate constant and the speed of the reaction. Nonetheless, increasing the temperature can also increase the charge per unit of the reaction. Does that mean that at extremely loftier temperature, enzymes can operate at farthermost speed?

Solutions

1. Use the Arrhenius Equation: \(chiliad = Ae^{-E_a/RT}\)

k is the rate constant, A is the pre-exponential factor, T is temperature and R is gas abiding (8.314 J/molK)
ln(xi) = (xx)e^{-Due east_a} ^{/(viii.314)(345)}
Due east_a = 6084.1 J/mol

2. Employ the equation: \( \ln \left (\dfrac{k_1}{k_2} \correct ) = \dfrac{-E_a}{R} \left(\dfrac{1}{T_1} - \dfrac{1}{T_2}\correct)\)

ln(33/45) = [-Ea/8.314](ane/298 - 1/675)
E_a = 1375.8 J/mol

3. Use the equation \(\Delta{1000} = \Delta{H} - T \Delta{S}\)

\(\Delta{G} = (34 \times 1000) - (334)(66)\)
\(\Delta{M} = 11956\, J/mol\)

4. Use the equation \(\ln k = \ln A - \dfrac{E_a}{RT}\) to calculate the activation energy of the forrard reaction

ln(fifty) = (30)due east^-East_a ^{/(viii.314)(679)}
E_a = 11500 J/mol
Considering the contrary reaction's activation energy is the activation energy of the forrad reaction plus ΔH of the reaction:
11500 J/mol + (23 kJ/mol Ten 1000) = 34500 J/mol

5. No. Most enzymes denature at high temperatures. At some point, the rate of the reaction and rate constant will subtract significantly and eventually drop to zero. Once the enzyme is denatured, the alternate pathway is lost, and the original pathway will take more time to complete.

References

Atkins P., de Paua J.. Physical Chemistry for the Life Sciences. pg 256-259. New York. Oxford Univeristy Printing. 2006.
Garrett R., Grisham C. Biochemistry. third Edition. pg 64. California. Thomson Learning, Inc. 2005
Wade L.G. Organic Chemistry. sixth Edition. pg 139-142. New Jersey. Pearson Prentice Hall. 2006.

Contributors and Attributions

Matthew Bui, Kan, Mentum Fung Kelvin, Sinh Le, Eva Tan