how to find magnitude of a vector

In physics, when yous're given the vector components, such as (three, 4), you can hands convert to the magnitude/bending fashion of expressing vectors using trigonometry.

For instance, take a look at the vector in the image.

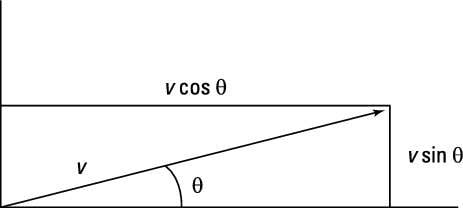
Suppose that you're given the coordinates of the end of the vector and want to observe its magnitude, v, and angle, theta. Because of your knowledge of trigonometry, you know

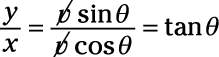
Where tan theta is the tangent of the bending. This means that

theta = tan^–1(y/x)

Suppose that the coordinates of the vector are (three, 4). You can find the bending theta equally the tan^–1(four/iii) = 53 degrees.

You can use the Pythagorean theorem to detect the hypotenuse — the magnitude, v — of the triangle formed by x, y, and v:

Plug in the numbers for this example to get

Then if you have a vector given past the coordinates (3, 4), its magnitude is 5, and its angle is 53 degrees.

Sample question

Catechumen the vector given by the coordinates (ane.0, 5.0) into magnitude/angle format.

The correct answer is magnitude 5.ane, angle 79 degrees.
1. Apply the Pythagorean theorem to find the magnitude. Plug in the numbers to get 5.1.
2. Apply the equation theta= tan^–i(y/x) to find the angle. Plug in the numbers to get tan^–i(5.0/1.0) = 79 degrees.

Practice questions

Catechumen the vector (5.0, 7.0) into magnitude/angle class.
Convert the vector (13.0, 13.0) into magnitude/angle form.
Convert the vector (–1.0, 1.0) into magnitude/angle course.
Convert the vector (–5.0, –7.0) into magnitude/angle form.

Post-obit are answers to the practice questions:

Magnitude eight.half dozen, angle 54 degrees
1. Apply the equation
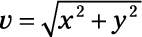
to detect the magnitude, which is 8.6.
1. Use the equation theta = tan^–1(y/ten) to find the angle: tan^–one(7.0/5.0) = 54 degrees.
Magnitude 18.4, angle 45 degrees
1. Utilise the equation
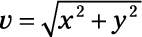
to find the magnitude, which is 18.4.
1. Apply the equation theta = tan^–1(y/x) to find the bending: tan^–1(13.0/13.0) = 45 degrees.
Magnitude 1.4, angle 135 degrees
1. Utilize the equation
  
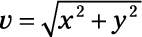
  to find the magnitude, which is 1.4.
2. Apply the equation theta = tan^–1(y/x) to find the bending: tan^–1(1.0/–1.0) = –45 degrees.
  
  All the same, note that the angle must really be betwixt 90 degrees and 180 degrees because the first vector component is negative and the second is positive. That ways you should add 180 degrees to –45 degrees, giving you 135 degrees (the tangent of 135 degrees is also 1.0/–1.0 = –1.0).
Magnitude viii.vi, angle 234 degrees
1. Employ the equation
  
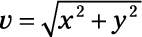
  to observe the magnitude, which is 8.vi.
2. Apply the equation theta = tan–1(y/10) to find the angle: tan–1(–7.0/–v.0) = 54 degrees.
  
  Nevertheless, annotation that the angle must really be between 180 degrees and 270 degrees because both vector components are negative. That means y'all should add together 180 degrees to 54 degrees, giving y'all 234 degrees (the tangent of 234 degrees is besides –vii.0/–5.0 = 7.0/5.0).